Replaceable Substructures for Efficient Part-Based Modeling
Résumé
A popular mode of shape synthesis involves mixing and matching parts from different objects to form a coherent whole. The key challenge is to efficiently synthesize shape variations that are plausible, both locally and globally. A major obstacle is to assemble the objects with local consistency, i.e., all the connections between parts are valid with no dangling open connections. The combinatorial complexity of this problem limits existing methods in geometric and/or topological variations of the synthesized models. In this work, we introduce replaceable substructures as arrangements of parts that can be interchanged while ensuring boundary consistency. The consistency information is extracted from part labels and connections in the original source models. We present a polynomial time algorithm that discovers such substructures by working on a dual of the original shape graph that encodes inter-part connectivity. We demonstrate the algorithm on a range of test examples producing plausible shape variations, both from a geometric and from a topological viewpoint.
Fichier principal
 shapeGraph.pdf (24.07 Mo)
Télécharger le fichier
shapeGraph.pdf (24.07 Mo)
Télécharger le fichier
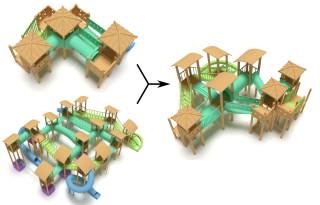 image2993.png (1.22 Mo)
Télécharger le fichier
image2993.png (1.22 Mo)
Télécharger le fichier
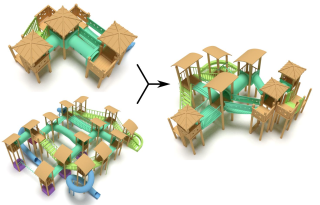 image2993.jpg (123.98 Ko)
Télécharger le fichier
image2993.jpg (123.98 Ko)
Télécharger le fichier
| Origine | Fichiers produits par l'(les) auteur(s) |
|---|
| Format | Figure, Image |
|---|---|
| Origine | Fichiers produits par l'(les) auteur(s) |
| Format | Figure, Image |
|---|---|
| Origine | Fichiers produits par l'(les) auteur(s) |
Loading...