ConTesse: Accurate Occluding Contours for Subdivision Surfaces
Résumé
This paper proposes a method for computing the visible occluding contours of subdivision surfaces. The paper first introduces new theory for contour visibility of smooth surfaces. Necessary and sufficient conditions are introduced for when a sampled occluding contour is valid, that is, when it may be assigned consistent visibility. Previous methods do not guarantee these conditions, which helps explain why smooth contour visibility has been such a challenging problem in the past. The paper then proposes an algorithm that, given a subdivision surface, finds sampled contours satisfying these conditions, and then generates a new triangle mesh matching the given occluding contours. The contours of the output triangle mesh may then be rendered with standard non-photorealistic rendering algorithms, using the mesh for visibility computation. The method can be applied to any triangle mesh, by treating it as the base mesh of a subdivision surface.
Fichier principal
 ConTesse.pdf (22.93 Mo)
Télécharger le fichier
ConTesse.pdf (22.93 Mo)
Télécharger le fichier
 bunny_000001_loop_qi_chained.png (121.35 Ko)
Télécharger le fichier
bunny_000001_loop_qi_chained.png (121.35 Ko)
Télécharger le fichier
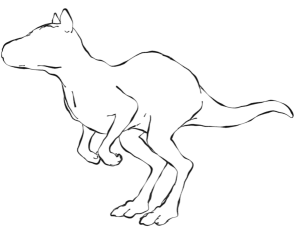 killeroo_small_cam212_topo_wedge.png (161.9 Ko)
Télécharger le fichier
killeroo_small_cam212_topo_wedge.png (161.9 Ko)
Télécharger le fichier
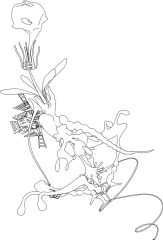 yeahright_220.png (122.37 Ko)
Télécharger le fichier
yeahright_220.png (122.37 Ko)
Télécharger le fichier
| Origine | Fichiers produits par l'(les) auteur(s) |
|---|
| Format | Figure, Image |
|---|
| Format | Figure, Image |
|---|
| Format | Figure, Image |
|---|