Inverse design of a suspended Kirchhoff rod: From theory to practice
Résumé
Our study focuses on finding the natural shape of a given hanging deformed isotropic rod, made of a
known material, the input shape is described as a mere geometric curve that we subsequently frame
to compute a material curvature field and feed our inverse problem. We prove that the natural shape
of the rod satisfying equilibrium exists and is unique, regardless of the infinity compatible frames
for the input curve. The natural shape is computed efficiently by solving in sequence three linear
initial value problems. We illustrate our theoretical results trough numerical examples of well known
curves to which we apply our inverse procedure. By direct simulation we show that indeed those
natural shapes fall, under the effect of gravity, onto the expected equilibrium. We stress on the fact
that the obtained rest shapes are complex and far from intuitive. Finally, we complement this study
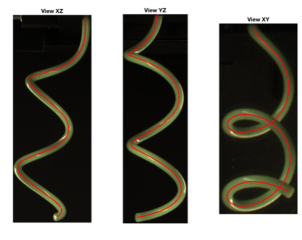
with experimental corroborations. By means of a standard array of cameras, we spatially reconstruct
real elastic hanging rods with well-defined geometrical features. We find some good agreements
with model prediction despite the experimental limitations on the estimation of the curvature fields
of the rod’s center line.
Fichier principal
 APS_2019_abstract.pdf (41)
Télécharger le fichier
APS_2019_abstract.pdf (41)
Télécharger le fichier
 APS2019.png (261)
Télécharger le fichier
APS2019.png (261)
Télécharger le fichier
| Origine | Fichiers produits par l'(les) auteur(s) |
|---|
| Format | Figure, Image |
|---|---|
| Licence |

