Matrix formulae for Resultants and Discriminants of Bivariate Tensor-product Polynomials
Résumé
The construction of optimal resultant formulae for polynomial systems is one of the main areas of research in computational algebraic geometry. However, most of the constructions are restricted to formulae for unmixed polynomial systems, that is, systems of polynomials which all have the same support. Such a condition is restrictive, since mixed systems of equations arise frequently in many problems. Nevertheless, resultant formulae for mixed polynomial systems is a very challenging problem. We present a square, Koszul-type, matrix, the determinant of which is the resultant of an arbitrary (mixed) bivariate tensor-product polynomial system. The formula generalizes the classical Sylvester matrix of two univariate polynomials, since it expresses a map of degree one, that is, the elements of the corresponding matrix are up to sign the coefficients of the input polynomials. Interestingly, the matrix expresses a primal-dual multiplication map, that is, the tensor product of a univariate multiplication map with a map expressing derivation in a dual space. In addition we prove an impossibility result which states that for tensor-product systems with more than two (affine) variables there are no universal degree-one formulae, unless the system is unmixed. Last but not least, we present applications of the new construction in the efficient computation of discriminants and mixed discriminants.
Fichier principal
 bmt-j-bivar.pdf (348)
Télécharger le fichier
bmt-j-bivar.pdf (348)
Télécharger le fichier
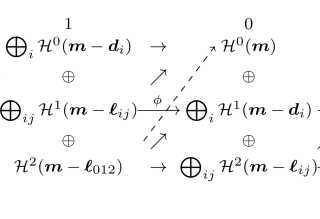 vignette.png (43)
Télécharger le fichier
vignette.png (43)
Télécharger le fichier
| Origine | Fichiers produits par l'(les) auteur(s) |
|---|
| Format | Figure, Image |
|---|
Loading...