Expected Length of the Voronoi Path in a High Dimensional Poisson-Delaunay Triangulation - Inria - Institut national de recherche en sciences et technologies du numérique
Article Dans Une Revue Discrete and Computational Geometry Année : 2018

Expected Length of the Voronoi Path in a High Dimensional Poisson-Delaunay Triangulation

Résumé

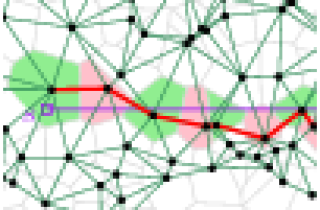
Let X be a d dimensional Poisson point process. We prove that the expected length of the Voronoi path between two points at distance 1 in the Delaunay triangulation associated with X is sqrt(2d/π) + O(d^(−1/2) when d → ∞. In any dimension, we also provide a precise interval containing the actual value; in 3D the expected length is between 1.4977 and 1.50007.

Domaines

Géométrie algorithmique [cs.CG]
Fichier principal
Vignette du fichier
paper.pdf (789.02 Ko) Télécharger le fichier
Vignette du fichier
vignette.png (14.78 Ko) Télécharger le fichier
maple-computations.zip (41.32 Ko) Télécharger le fichier
Origine Fichiers produits par l'(les) auteur(s)
Format Figure, Image
Origine Fichiers produits par l'(les) auteur(s)
Origine Fichiers produits par l'(les) auteur(s)

Olivier Devillers  : Connectez-vous pour contacter le contributeur

https://inria.hal.science/hal-01477030

Soumis le : dimanche 26 février 2017-19:32:49

Dernière modification le : jeudi 5 décembre 2024-03:21:55

Archivage à long terme le : samedi 27 mai 2017-12:33:05

Télécharger pour visualiser

Dates et versions

hal-01477030 , version 1 (26-02-2017)

Identifiants

Citer

Pedro Machado Manhães de Castro, Olivier Devillers. Expected Length of the Voronoi Path in a High Dimensional Poisson-Delaunay Triangulation. Discrete and Computational Geometry, 2018, 60 (1), pp.200--219. ⟨10.1007/s00454-017-9866-y⟩. ⟨hal-01477030⟩

Exporter

BibTeX XML-TEI Dublin Core DC Terms EndNote DataCite

Collections

CNRS INRIA UNIV-LORRAINE INRIA2 LORIA LORIA-ALGO
315 Consultations
232 Téléchargements

Altmetric

Partager

More