Transition probabilities for piecewise affine models of genetic networks
Résumé
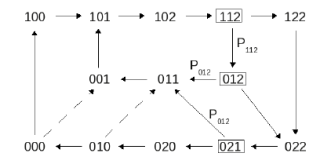
In the piecewise affine framework, trajectories evolve among hyperrectangles in the state space. A qualitative description of the dynamics - useful for models of genetic networks - can be obtained by viewing each hyperrectangle as a node in a discrete system, so that trajectories follow a path in a transition graph. In this paper, a probabilistic interpretation is given for the transition between two nodes A and B, based on the volume of the initial conditions on hyperrectangle A whose trajectories cross to B. In an example consisting of two intertwinned negative loops, this probabilistic interpretation is used to predict the most likely periodic orbit given a set of parameters, or to find parameters such that the system yields a desired periodic orbit with a high probability.
Domaines
Modélisation et simulation
Fichier principal
 ChavesFarcotGouze-MTNS10.pdf (214.09 Ko)
Télécharger le fichier
ChavesFarcotGouze-MTNS10.pdf (214.09 Ko)
Télécharger le fichier
 basilic_image_mtns10.png (8.18 Ko)
Télécharger le fichier
basilic_image_mtns10.png (8.18 Ko)
Télécharger le fichier
| Origine | Fichiers produits par l'(les) auteur(s) |
|---|
| Format | Figure, Image |
|---|
Loading...