Probabilistic approach for predicting periodic orbits in piecewise affine differential models
Résumé
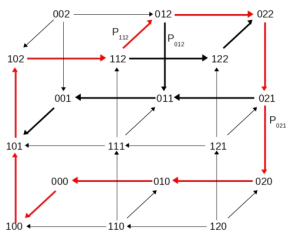
Piecewise affine models provide a qualitative description of the dynamics of a system, and are often used to study genetic regulatory networks. The state space of a piecewise affine system is partitioned into hyperrectangles, which can be represented as nodes in a directed graph, so that the system's trajectories follow a path in a transition graph.This paper proposes and compares two definitions of probability of transition between two nodes A and B of the graph, based on the volume of the initial conditions on the hyperrectangle A whose trajectories cross to B. The parameters of the system can thus be compared to the observed transitions between two hyperrectangles. This property may become useful to identify sets of parameters for which the system yields a desired periodic orbit with a high probability, or to predict the most likely periodic orbit given a set of parameters, as illustrated by a gene regulatory system composed of two intertwined negative loops.
Domaines
Modélisation et simulation
Fichier principal
 chaves-farcot-gouze-bmb2013.pdf (269.91 Ko)
Télécharger le fichier
chaves-farcot-gouze-bmb2013.pdf (269.91 Ko)
Télécharger le fichier
 temp4.jpg (58.97 Ko)
Télécharger le fichier
temp4.jpg (58.97 Ko)
Télécharger le fichier
| Origine | Fichiers produits par l'(les) auteur(s) |
|---|
| Format | Figure, Image |
|---|---|
| Origine | Fichiers produits par l'(les) auteur(s) |
Loading...