Progressive Discrete Domains for Implicit Surface Reconstruction
Résumé
Many global implicit surface reconstruction algorithms formulate the problem as a volumetric energy minimization, trading data fitting for geometric regularization. As a result, the output surfaces may be located arbitrarily far away from the input samples. This is amplified when considering i) strong regularization terms, ii) sparsely distributed samples or iii) missing data. This breaks the strong assumption commonly used by popular octree-based and triangulation-based approaches that the output surface should be located near the input samples. As these approaches refine during a pre-process, their cells near the input samples, the implicit solver deals with a domain discretization not fully adapted to the final isosurface.We relax this assumption and propose a progressive coarse-to-fine approach that jointly refines the implicit function and its representation domain, through iterating solver, optimization and refinement steps applied to a 3D Delaunay triangulation. There are several advantages to this approach: the discretized domain is adapted near the isosurface and optimized to improve both the solver conditioning and the quality of the output surface mesh contoured via marching tetrahedra.
Fichier principal
 sgp2021.pdf (57.24 Mo)
Télécharger le fichier
sgp2021.pdf (57.24 Mo)
Télécharger le fichier
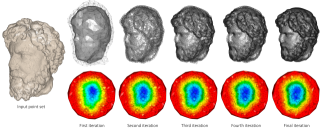 poseidon.png (3.04 Mo)
Télécharger le fichier
poseidon.png (3.04 Mo)
Télécharger le fichier
| Origine | Fichiers produits par l'(les) auteur(s) |
|---|
| Format | Figure, Image |
|---|